Let us first define the type of quantum dot (QD) to be modeled: a self-assembled semiconductor structure having cylindrical symmetry, that confines both electrons and holes and can be excited by light. Furthermore, the confinement is stronger in $z$ and we can restrict the study to the dynamics in the perpendicular plane. We will call this structure a disk-shaped quantum dot (DSQD), and study the excitation of electron-heavy hole pairs.
In the post on electronic states we mentioned that the nanostructure's wavefunction is comes from replacing the phase $\exp(-i\mathbf k \cdot \mathbf r)$ by the corresponding envelope function that has the symmetry of the nanoparticle. In the case of the QD
$$
\psi(\mathbf r, \theta, z) = \phi(r,\theta) Z(z) u(\mathbf r)
$$
where, because of the assumed shape of the dot, it is convenient to use cylindrical coordinates $\{ r, \theta, z\}$ for the envelope functions $\phi$ and $Z$; $u(\mathbf r)$ is the periodic Bloch part. The angular function is
$$
\phi(r,\theta)
=
N \, e^{-[r/(2 r_0)]^2} L_s^n(r^2/r_0^2) \left(\frac{r}{r_0}\right)^{|n|} e^{-i n \theta}
~~~~~~~~ (1)
$$
with $N$ a normalization constant, $L_s^n(x)$ is the generalized Laguerre polynomial, and $r_0$ is the size of the electron's wavefunction in the dot. Because of our assumptions above, electrons will always remain in the same state $Z(z)$, and so it is unnecessary to specify this function.
Due to the functional form of Eq. (1) we choose to work with Laguerre-Gaussian beams instead of Bessel beams. Laguerre-Gaussian beams are very popular in the twisted-light literature -in fact more than Bessel beams. They are easily prepared in the lab from Hermite-Gaussian beams using a variety of techniques (see the post on beam generation), but they are solutions to Maxwell's equations in the paraxial approximation, in contrast to Bessel beams that are solutions to the full equations. The vector potential of a Laguerre-Gaussian beam is given by
$$
\mathbf A(\mathbf r,t)
=
{\boldsymbol{\epsilon}} \, N' e^{-[r/w_0]^2} L_s^n(2 r^2/w_0^2) \left(\frac{r}{2 w_0}\right)^{|\ell|} e^{-i \ell \theta}
~~~~~~~~ (2)
$$
where $\boldsymbol{\epsilon}$ is the polarization vector.
The similarities between Eqs. (1) and (2) allow for nice analytical solutions. We simply want to calculate matrix elements of $H_I=-(q/m) \mathbf A(\mathbf r,t) \cdot \mathbf p$ to determine the possible optical transitions induced by twisted light [2]. The matrix elements can then be used in different ways, e.g. in the Fermi's Golden Rule, as building blocks in equations of motion, etc. The details of the calculation are given in Ref. [1]; the resulting matrix element is
$$
\langle{csn}|H_I|{vtm}\rangle
=
C \, e^{-i \omega t} (\boldsymbol{\epsilon} \cdot \mathbf p_{cv})
\delta_{\ell,m-n} \,
I(\sqrt{2} r_0/w_0)
~~~~~~~~ (3)
$$
where $C$ is a constant and
\begin{eqnarray}
I(\sqrt{2} r_0/w_0)
&=&
\left(\frac{\sqrt{2} r_0}{w_0}\right)^{|\ell|}
\int_0^\infty dx \, x^{(|n|+|m|+|\ell|)/2} \exp[{-x [1+(r_0/\sqrt{2}w_0)^2]}]
\\
&&
\times
L_p^\ell(\left(\sqrt{2} r_0/w_0\right)^2 x ) L_t^m(x) L_s^n(x)
\end{eqnarray}
and $x=r/(\sqrt{2}r_0)$.
Optical transitions
The similarities between Eqs. (1) and (2) allow for nice analytical solutions. We simply want to calculate matrix elements of $H_I=-(q/m) \mathbf A(\mathbf r,t) \cdot \mathbf p$ to determine the possible optical transitions induced by twisted light [2]. The matrix elements can then be used in different ways, e.g. in the Fermi's Golden Rule, as building blocks in equations of motion, etc. The details of the calculation are given in Ref. [1]; the resulting matrix element is
$$
\langle{csn}|H_I|{vtm}\rangle
=
C \, e^{-i \omega t} (\boldsymbol{\epsilon} \cdot \mathbf p_{cv})
\delta_{\ell,m-n} \,
I(\sqrt{2} r_0/w_0)
~~~~~~~~ (3)
$$
where $C$ is a constant and
\begin{eqnarray}
I(\sqrt{2} r_0/w_0)
&=&
\left(\frac{\sqrt{2} r_0}{w_0}\right)^{|\ell|}
\int_0^\infty dx \, x^{(|n|+|m|+|\ell|)/2} \exp[{-x [1+(r_0/\sqrt{2}w_0)^2]}]
\\
&&
\times
L_p^\ell(\left(\sqrt{2} r_0/w_0\right)^2 x ) L_t^m(x) L_s^n(x)
\end{eqnarray}
and $x=r/(\sqrt{2}r_0)$.
Optical transitions
Inspecting Eq. (3) and $I(\sqrt{2} r_0/w_0)$ we first notice that the (circular) polarization $\boldsymbol{\epsilon}$ relates to the microscopic matrix element of the linear momentum operator $\langle u_c|\mathbf p|u_v\rangle$ [3], exactly as in the case of excitation by plane wave. Therefore, twisted light does not change the selection rule that determines which bands will the light connect, in particular it is the polarization (or photon spin angular momentum) that fixes what is the spin of the electron excited to the conduction band. To reinforce this idea, note that the spatial variation of the light field appears only in $I(\sqrt{2} r_0/w_0)$, because it is smooth with respect to the dimensions of the semiconductor unit cell.
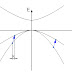
The delta function $\delta_{\ell,m-n}$ is an additional selection rule imposed by twisted light on the macroscopic motion of electrons, telling us that the transitions are not vertical and that electrons absorb the orbital angular momentum carried by the light field. This process is depicted in Fig. 1.
The quantity $I(\sqrt{2} r_0/w_0)$ contains information on the relative sizes of beam and dots, and the radial structure of the field and electron's wavefunction. The most important part is perhaps the factor $\left(\sqrt{2} r_0/w_0\right)^{|\ell|}$, that points to the fact that twisted light has a vanishing amplitude on axis (the optical vortex): small QDs will only weakly interact with the light beam, and the interaction strength decreases with larger $\ell$. Since the integral also contains the ratio $\sqrt{2} r_0/w_0$, one may wonder what is its functional dependence.
A relevant example is that of transition from the uppermost valence state. For small QD we can solved the integral analytically, and compare the matrix elements of excitation by plane waves (PW) and twisted light (TL)
$$
{\langle c|H_I|_{TL}|v \rangle \over \langle c|H_I|_{PW}|v\rangle}
\propto
\left(\frac{\sqrt{2}\, r_0}{w_0}\right)^{|\ell|}
$$
[the ratio is between the PW and the TL -to $(0)$- transitions showed in Figure 1].
The delta function $\delta_{\ell,m-n}$ is an additional selection rule imposed by twisted light on the macroscopic motion of electrons, telling us that the transitions are not vertical and that electrons absorb the orbital angular momentum carried by the light field. This process is depicted in Fig. 1.
 |
| Figure 1: Transitions induced by plane waves and twisted light with $\ell = 1$ on a quantum dot. The number in between parenthesis is the radial quantum number $s$. Twisted light produces a non-vertical transition, i. e. moves an electron from $n=0$ to $n=1$. |
A relevant example is that of transition from the uppermost valence state. For small QD we can solved the integral analytically, and compare the matrix elements of excitation by plane waves (PW) and twisted light (TL)
$$
{\langle c|H_I|_{TL}|v \rangle \over \langle c|H_I|_{PW}|v\rangle}
\propto
\left(\frac{\sqrt{2}\, r_0}{w_0}\right)^{|\ell|}
$$
[the ratio is between the PW and the TL -to $(0)$- transitions showed in Figure 1].
It is also possible to study numerically what happens for arbitrary initial state and arbitrary ratio of QD to beam size, see Ref. [1].
To summarize, a single pulse of twisted light can control more effectively electronic states in QDs, producing a variety of electron-hole pairs. However, the coupling constant for the interaction of a paraxial beam of twisted light and small QDs is rather small, and would require intense fields and long irradiation times. This drawback can be partially circumvented with the use of highly focus (nonparaxial) beams of twisted light, larger nanostructures, etc.
References
[1] G. F. Quinteiro and P. I. Tamborenea, Electronic transitions in disk-shaped quantum dots induced by twisted light, Phys. Rev. B 79, 155450 (2009).
[2] Instead of the Coulomb gauge interaction $\mathbf A \cdot \mathbf p$, one can use a Hamiltonian in terms of electric fields of the type $\mathbf E \cdot \mathbf r$. The predictions described in this post regarding old and new selection rules and the dependence of intensity on the ratio $r_0/w_0$ are the same in both gauges, as will be shown in a future post.
[3] One can think of $p_{vc}$ as proportional to the matrix element of the dipole $q r_{vc}$.
0 comments:
Post a Comment